FM Spectrum Graphs

Bessel Graph

Note - Negative amplitudes are also shown as positive dotted lines (This gives a better picture of the harmonic strengths generated). Negative amplitudes denote phase inversion.

The graph is scaled for a DX-7 range. The DX-7 Modulator Output is shown in the upper-right corner. The equivalent Modulation Index is shown in the bottom right-corner. The slider-indicator on the right is scaled for a DX-7 Modulator Operator (just another tool to help visualise the process).

The spectrums displayed are M:C=1:16, M:C=1:8, M:C=1:1, M:C=2:1 and M:C=3:1 (from left to right). The first four graphs have coincident reflected sidebands while the last does not. Inverted phase frequencies are shown as dotted lines. Output values are for DX-7 series of synths.

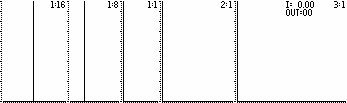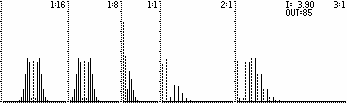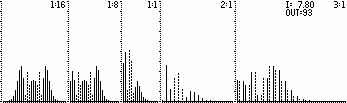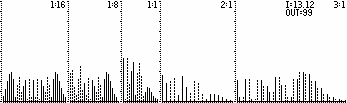
• M:C = 1:16 ~ This is a good example of the FM spectrum without the interference of reflected sidebands. It shows the basic spread of sidebands as modulation is increased. C=16 is high relative to M=1. In this case, LS#16 (the 16th lower sideband) is at F1, LS#17 is at F0 (ie silent) and LS#18 is inverted at F1 (LS#18 is the first refected sideband). The sidebands spread out on both sides of "C" symmetrically. The effect of reflected sidebands (ie LS#18 onwards) only occurs at high modulation amounts.
• M:C = 1:8 ~ This example also shows the symmetrical spread but has some interference of coincident reflected sidebands. C=8 is medium relative to M=1. In this case, LS#8 is at F1, LS#9 is at F0 (silent) and LS#10 is inverted at F1 (LS#10 is the first reflected sideband). The sidebands spread out symmetrically up to a point at moderate modulation amounts where the effect of reflected sidebands (ie LS#10 onwards) occurs.
• MC = 1:1 ~ This example shows the dramatic effect of the interference of coincident reflected sidebands. C=1 is low relative to M=1 (a very popular ratio for string sounds). In this case, LS#1 is at F0 (silent) and LS#2 is inverted at F1 (LS#2 is the first reflected sideband). The effect of reflected sidebands (ie LS#2 onwards) occurs at low modulation amounts.
• M:C = 2:1 ~ Another example of the effect of the interference of coincident reflected sidebands. C=1 is low relative to M=2 (a ratio for wind instruments perhaps). In this case, LS#1 is inverted at F1 (the first lower sideband is already reflected). The effect of reflected sidebands (ie LS#1 onwards) occurs at low modulation amounts.
• M:C = 3:1 ~ This is a good example of the effect of non-coincident reflected sidebands. C=1 is low relative to M=3 (perhaps for wood percussion sounds). In this case, LS#1 is inverted at F2 (the first lower sideband is already reflected) but is not coincident with another sideband. The effect of reflected sidebands (ie LS#1 onwards) occurs at low modulation amounts.